Plates/Shells - Results

When the model is solved, there are several groups of results spreadsheets specifically for the plates. 

Plate Stress Results 

To access the Plate Principal Stresses results spreadsheet:

1. Click on Results in the Explorer panel to expand Env (Envelope) results.

2. Click on Plate Stresses to open the Plate Stresses spreadsheet.

   

   Click on image to enlarge it

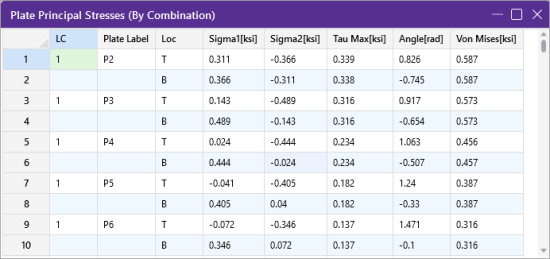
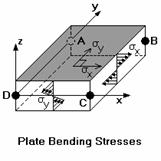
The plate stresses are listed for the top and bottom of each active plate. The principal stresses sigma1 (σ₁) and sigma2 (σ₂) are the maximum and minimum normal stresses on the element at the geometric center of the plate. The Tau Max (t_max) stress is the maximum shear stress. The Angle entry is the angle between the element's local x-axis, and the direction of the σ₁ stress (in radians). The Von Mises value is calculated using σ₁ and σ₂, but not σ₃ which isn't available for a surface (plate/shell) element, so this Von Mises stress does not include any transverse shear forces.

The equations are:

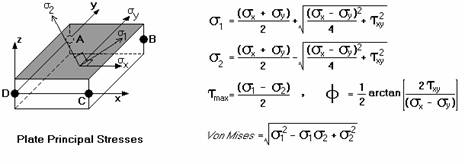
The angle, Φ, is the angle in radians between the maximum normal stress and the local x-axis. The direction of the maximum shear stress, t_max, is  ±  π/4 radians from the principal stress directions.

The Von Mises stress is a combination of the principal stresses and represents the maximum energy of distortion within the element. This stress can be compared to the tensile yield stress of ductile materials for design purposes. For example, if a steel plate has a tensile yield stress of 36ksi, then a Von Mises stress of 36ksi or higher would indicate yielding of the material at some point in the plate.

The σ_x , σ_y , and σ_xy values used to calculate the stresses are a combination of the plate bending and membrane stresses, thus the results are listed for the top and bottom surfaces of the element. The “Top” is the extreme fiber of the element in the positive local z direction, and the “Bottom” is the extreme fiber of the element in the negative local z direction. The membrane stresses are constant through the thickness of the element, while the bending stresses vary through the thickness of the element, very similar to the bending stress distribution in a beam.

For enveloped results the maximum and minimum value at each location is listed. The load combination producing the maximum or minimum is also listed, in the "LC" column.

Plate Force Results

To access the Plate Forces spreadsheet:

1. Click on Results in the Explorer panel to expand Env (Envelope) results.

2. Click on Plate Stresses to open the Plate Stresses spreadsheet.

   

   Click on image to enlarge it

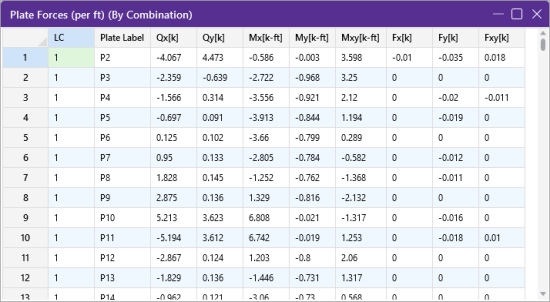
The Plate Forces are listed for each active plate. Interpretation of output results is perhaps the most challenging aspect in using the plate/shell element. The results for the plates are shown for the geometric center of the plate.

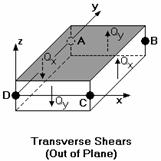
The forces (Q_x and Q_y) are the out-of-plane (also called “transverse”) shears that occur through the thickness of the element. The Q_x shear occurs on the element faces that are perpendicular to the local x-axis, and the Q_y shear occurs on the element faces that are perpendicular to the local y-axis. Q_x is positive in the z-direction on the element face whose normal vector is in the positive x-direction. This is also the σ_x face. Q_y is positive in the z-direction on the element face whose normal vector is in the positive y-direction. This is also the σ_y face. The total transverse shear on an element face is found by multiplying the given force by the width of the element face.

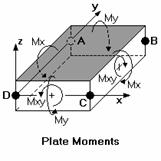
The plate bending moments (M_x, M_y and M_xy) are the plate forces that induce linearly varying bending stresses through the thickness of the element. Mx is the moment that causes stresses in the positive x-direction on the top of the element. Likewise, M_y is the moment that causes stresses in the positive y-direction on the top of the element. M_x can then be thought of as occurring on element faces that are perpendicular to the local x-axis, and the M_y moment occurs on faces that are perpendicular to the local y axis. To calculate the total M_x or M_y on the face of an element, multiply the given value by the length of the element that is parallel to the axis of the moment. For example, looking at the 'Plate Moments' figure above, the total M_x moment could be obtained by multiplying the given M_x force by the length of side BC (the distance from joint B to joint C). The total M_y force can be calculated in the same way by instead using the length of side DC.

The M_xy moment is the out-of-plane twist or warp in the element. This moment can be added to the M_x or M_y moment to obtain the 'total' M_x or M_y moment in the element for design purposes. This direct addition is valid since on either the top or bottom surface, the bending stresses from M_xy will be going in the same direction as the M_x and M_y moments.

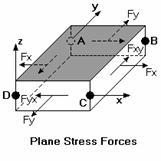
The plane stress forces (F_x , F_y and F_xy) are those forces that occur in the plane of the plate. These forces, which are also called “membrane” forces, are constant through the thickness of the element. F_x and F_y are the normal forces that occur respectively in the direction of the local plate x and y-axes, positive values indicating tension. These forces are reported as a force/unit length. To get the total force on an element, you would need to multiply the given value by the length of the element that is perpendicular to the normal force. For example, looking at the 'Plane Stress Forces' figure, the total Fx force could be obtained by multiplying the given F_x force by the length of side BC (the distance from joint B to joint C).

The Fxy force is the in-plane shear force that occurs along the side of the element. The subscript 'xy' indicates that the shear occurs on the face of the element that is perpendicular to the x-axis and is pointing in the y-direction. Fyx is the complementary shear force, where the subscript 'yx' indicates that the shear occurs on the face of the element that is perpendicular to the y-axis and is pointing in the x-direction. RISA-3D only gives values for Fxy because Fxy and F_yx are numerically equal. The total in-plane shear can be obtained by multiplying the given force value by the  length of the element that is parallel to the shear force. For example, when looking at the 'Plane Stress Forces' figure, the total F_xy force which is parallel to the local y-axis could be obtained by multiplying the given F_xy force by the length of side BC.

Note that the plate bending (Q_x, Q_y, M_x, M_y, M_xy) and membrane (F_x, F_y, F_xy) results are forces per unit length. For example, a rectangular element with a B to C length of 10 feet showing a F_x force of 20K would have a total normal force on the B-C face of the element of 20K (per foot) times 10 feet, or 200K.

For enveloped results the maximum and minimum value is listed. The load combination producing the maximum or minimum is also listed, in the "LC" column.

Plate Corner Force Results 

To access the Plate Corner Forces spreadsheet:

1. Click on Results in the Explorer panel to expand Env (Envelope) results.

2. Click on Plate Stresses to open the Plate Stresses spreadsheet.

   

   Click on image to enlarge it

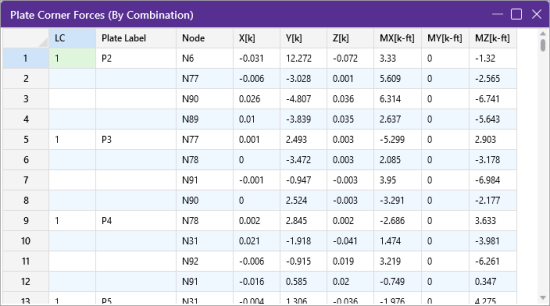
The plate corner forces are the global forces at the corner of each plate and are listed for each active plate.

These are the forces and moments calculated at the corners of the plates, in the GLOBAL directions. These values are obtained by multiplying the plate's corner displacements with the global stiffness matrix. Unlike the local stresses and forces, which are very accurate approximations, these corner forces represent EXACT results based on linear elastic theory. Also, the local forces are listed on a 'per unit length' basis, whereas these global direction corner forces represent the total force on the plate at the corner in the given direction, in the same way that joint reactions are reported. At any given joint, the corner forces for all plates connected to that joint should sum to zero (a requirement of equilibrium), assuming no members or boundary conditions are also present at the joint.

As an example of how to use these corner forces, you can obtain the total shear at a given level in a shear wall by adding the proper corner forces for the plates at that level. See Plate Modeling Examples to learn how to use the plate corner forces to get shear wall story shears and moments, as well as slab moments and shears.

For enveloped results the maximum and minimum value is listed. The load combination producing the maximum or minimum is also listed, in the "lc" column.